Piecewise functions. Piecewise function
Charts piecewise given functions
Murzalieva T.A. mathematics teacher MBOU "Bor Secondary secondary school» Boksitogorsk district Leningrad region
Target:
- master the linear spline method for constructing graphs containing a module;
- learn to apply it in simple situations.
Under spline(from the English spline - plank, rail) is usually understood as a piecewise given function.
Such functions have been known to mathematicians for a long time, starting with Euler (1707-1783, Swiss, German and Russian mathematician), but their intensive study began, in fact, only in the middle of the 20th century.
In 1946, Isaac Schoenberg (1903-1990, Romanian and American mathematician) first time using this term. Since 1960 with development computer technology the use of splines in computer graphics and modeling began.
1. Introduction
2. Definition of a linear spline
3. Module Definition
4. Graphing
One of the main purposes of functions is to describe real processes occurring in nature.
But for a long time, scientists - philosophers and natural scientists - have identified two types of processes: gradual ( continuous ) And spasmodic.
When a body falls to the ground, it first occurs continuous increase driving speed , and at the moment of collision with the surface of the earth speed changes abruptly , becoming equal to zero or changing the direction (sign) when the body “bounces” from the ground (for example, if the body is a ball).
But since there are discontinuous processes, then means of describing them are needed. For this purpose, functions are introduced that have ruptures .
a - by the formula y = h(x), and we will assume that each of the functions g(x) and h(x) is defined for all values of x and has no discontinuities. Then, if g(a) = h(a), then the function f(x) has a jump at x=a; if g(a) = h(a) = f(a), then the “combined” function f has no discontinuities. If both functions g and h are elementary, then f is called piecewise elementary. "width="640" - One way to introduce such discontinuities is next:
Let function y = f(x)
at x is defined by the formula y = g(x),
and when xa - formula y = h(x), and we will consider that each of the functions g(x) And h(x) is defined for all values of x and has no discontinuities.
Then , If g(a) = h(a), then the function f(x) has at x=a jump;
if g(a) = h(a) = f(a), then the "combined" function f has no breaks. If both functions g And h elementary, That f is called piecewise elementary.
Graphs of Continuous Functions
Graph the function:
Y = |X-1| + 1
X=1 – formula change point
Word "module" comes from the Latin word “modulus”, which means “measure”.
Modulus of numbers A called distance (in single segments) from the origin to point A ( A) .
This definition reveals geometric meaning module.
Module (absolute value) real number A the same number is called A≥ 0, and opposite number -A, if a
0 or x=0 y = -3x -2 at x "width="640" Graph the function y = 3|x|-2.
By definition of the modulus, we have: 3x – 2 at x0 or x=0
-3x -2 at x
x n) "width="640" . Let x be given 1 X 2 X n – points of change of formulas in piecewise elementary functions.
A function f defined for all x is called piecewise linear if it is linear on each interval
and besides, the coordination conditions are met, that is, at the points of changing formulas, the function does not suffer a break.
Continuous piecewise linear function called linear spline . Her schedule There is polyline with two infinite extreme links – left (corresponding to the values x n ) and right ( corresponding values x x n )
A piecewise elementary function can be defined by more than two formulas
Schedule - broken line with two infinite extreme links - left (x1).
Y=|x| - |x – 1|
Formula change points: x=0 and x=1.
Y(0)=-1, y(1)=1.
It is convenient to plot the graph of a piecewise linear function, pointing on the coordinate plane vertices of the broken line.
In addition to building n vertices should build Also two points : one to the left of the vertex A 1 ( x 1; y ( x 1)), the other - to the right of the top An ( xn ; y ( xn )).
Note that a discontinuous piecewise linear function cannot be represented as a linear combination of the moduli of binomials .
Graph the function y = x+ |x -2| - |X|.
A continuous piecewise linear function is called a linear spline
1.Points for changing formulas: X-2=0, X=2 ; X=0
2. Let's make a table:
U( 0 )= 0+|0-2|-|0|=0+2-0= 2 ;
y( 2 )=2+|2-2|-|2|=2+0-2= 0 ;
at (-1 )= -1+|-1-2| - |-1|= -1+3-1= 1 ;
y( 3 )=3+|3-2| - |3|=3+1-3= 1 .
Construct a graph of the function y = |x+1| +|x| – |x -2|.
1 .Points for changing formulas:
x+1=0, x=-1 ;
x=0 ; x-2=0, x=2.
2 . Let's make a table:
y(-2)=|-2+1|+|-2|-|-2-2|=1+2-4=-1;
y(-1)=|-1+1|+|-1|-|-1-2|=0+1-3=-2;
y(0)=1+0-2=-1;
y(2)=|2+1|+|2|-|2-2|=3+2-0=5;
y(3)=|3+1|+|3|-|3-2|=4+3-1=6.
|x – 1| = |x + 3|
Solve the equation:
Solution. Consider the function y = |x -1| - |x +3|
Let's build a graph of the function /using the linear spline method/
- Formula change points:
x -1 = 0, x = 1; x + 3 =0, x = - 3.
2. Let's make a table:
y(- 4) =|- 4–1| - |- 4+3| =|- 5| - | -1| = 5-1=4;
y( -3 )=|- 3-1| - |-3+3|=|-4| = 4;
y( 1 )=|1-1| - |1+3| = - 4 ;
y(-1) = 0.
y(2)=|2-1| - |2+3|=1 – 5 = - 4.
Answer: -1.
1. Construct graphs of piecewise linear functions using the linear spline method:
y = |x – 3| + |x|;
1). Formula change points:
2). Let's make a table:
2. Construct graphs of functions using the teaching aid “Live Mathematics” »
A) y = |2x – 4| + |x +1|
1) Formula change points:
2) y() =
B) Build function graphs, establish a pattern :
a) y = |x – 4| b) y = |x| +1
y = |x + 3| y = |x| - 3
y = |x – 3| y = |x| - 5
y = |x + 4| y = |x| + 4
Use the Point, Line, and Arrow tools on the toolbar.
1. “Charts” menu.
2. “Build a graph” tab.
.3. In the “Calculator” window, set the formula.
Graph the function:
1) Y = 2x + 4
1. Kozina M.E. Mathematics. Grades 8-9: collection of elective courses. – Volgograd: Teacher, 2006.
2. Yu. N. Makarychev, N. G. Mindyuk, K. I. Neshkov, S. B. Suvorova. Algebra: textbook. For 7th grade. general education institutions / ed. S. A. Telyakovsky. – 17th ed. – M.: Education, 2011
3. Yu. N. Makarychev, N. G. Mindyuk, K. I. Neshkov, S. B. Suvorova. Algebra: textbook. For 8th grade. general education institutions / ed. S. A. Telyakovsky. – 17th ed. – M.: Education, 2011
4. Wikipedia, the free encyclopedia
http://ru.wikipedia.org/wiki/Spline
7
Algebra lesson in grade 9A by teacher Mikitchuk Zh.N. Municipal educational institution "Secondary school No. 23"03/19/07Lesson topic: "Piecewise defined functions"
Goals:
- generalize and improve the knowledge, skills and abilities of students on the specified topic; to cultivate in students attentiveness, concentration, perseverance, and confidence in their knowledge; develop thinking abilities, logical thinking; speech culture, ability to apply theoretical knowledge.
- the concept of a piecewise given function; formulas of various functions, corresponding names and images of graphs;
- build a graph of a piecewise given function; read the chart; define a function analytically using a graph.
Lesson progress
I. Organizational and psychological moment. Let’s start our lesson with the words of D.K. Fadeev “Whatever problem you solve, at the end a happy moment awaits you - a joyful feeling of success, strengthening faith in your strength. Let these words gain real confirmation in our lesson. II. Checking homework. Let's start the lesson as usual with checking the d/z. - Repeat the definition of a piecewise function and the plan for studying functions. 1). On the board draw the graphs of piecewise functions you have invented (Fig. 1, 2, 3)2). Cards.№1. Arrange the order of studying the properties of functions:- convex; even, odd; range; limitation; monotone; continuity; the largest and smallest value of a function; domain of definition.
A) y = kx + b, k0; B) y = kx, k0;
B) y = , k0.
3).Oral work . – 2min
- Which function is called piecewise?
- What functions do the piecewise functions shown in Fig. 1, 2, 3 consist of? What other function names do you know? What are the graphs of the corresponding functions called? Is the figure shown in Fig. 4 a graph of any function? Why?
- repeat the steps of constructing a piecewise given function; apply generalized knowledge when solving non-standard problems.
The main topic of 8th grade is quadratic function, simulating uniformly accelerated processes. Example: the formula you studied in 9th grade for determining the resistance of a heated lamp (R) at constant power (P) and changing voltage (U). FormulaR =
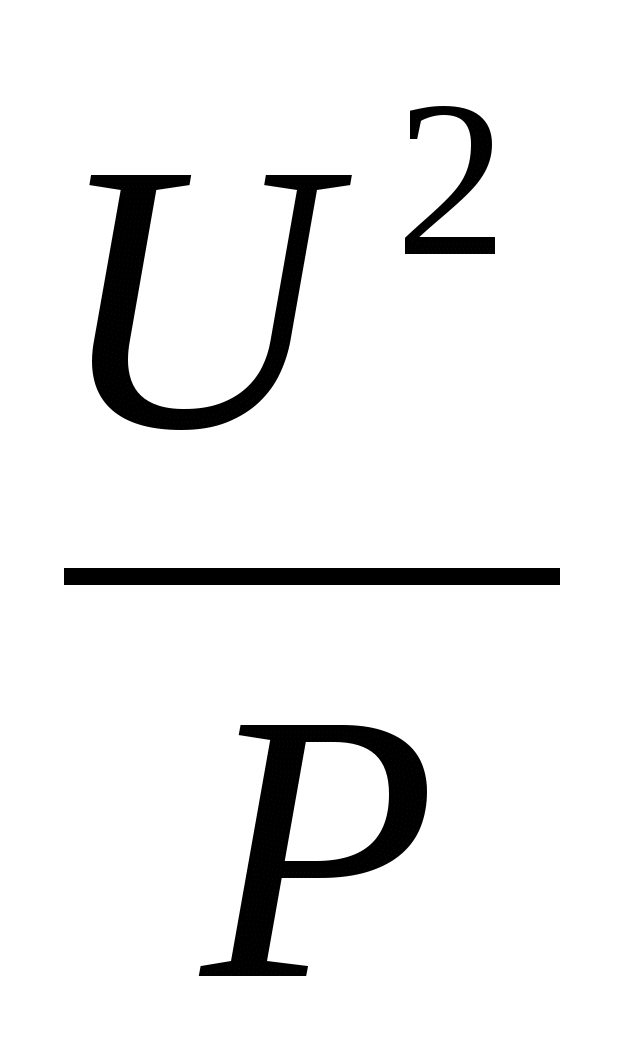, the graph is a branch of a parabola located in the first quarter. Throughout three years our knowledge about functions was enriched, the number of functions studied grew, and the set of tasks for solving which we had to resort to graphs was expanded. Name these types of tasks... - solving equations;- solving systems of equations;- solving inequalities;- study of the properties of functions.V. Preparing students for generalization activities. Let's remember one of the types of tasks, namely, studying the properties of functions or reading a graph. Let's turn to the textbook. Page 65 Fig. 20a from No. 250. Exercise: read the graph of the function. The procedure for studying the function is before us. 1. domain of definition – (-∞; +∞)2. even, odd - neither even nor odd3. monotony - increases [-3; +∞), decreases[-5;-3], constant (-∞; -5];4. boundedness – limited from below5. the largest and smallest value of the function – y max = 0, y max – does not exist;6. continuity - continuous throughout the entire domain of definition;7. The range of values is convex both down and up (-∞; -5] and [-2; +∞).VI. Reproduction of knowledge at a new level. You know that the construction and study of graphs of piecewise given functions are covered in the second part of the algebra exam in the functions section and are assessed with 4 and 6 points. Let's turn to the collection of tasks. Page 119 - No. 4.19-1). Solution: 1).y = - x, - quadratic function, graph - parabola, branches down (a = -1, a 0). x -2 -1 0 1 2 y -4 -1 0 1 4 2) y = 3x – 10, - linear function, graph - straightLet's make a table of some valuesx 3
3
y 0 -1 3) y= -3x -10, - linear function, graph - straightLet's make a table of some values x -3 -3 y 0 -1 4) Let's construct graphs of functions in one coordinate system and select parts of the graphs at given intervals.Let us find from the graph at what values of x the values of the function are non-negative. Answer: f(x) 0 at x = 0 and at
3 VII.Work on non-standard tasks.
No. 4.29-1), page 121. Solution: 1) Straight line (left) y = kx + b passes through the points (-4;0) and (-2;2). This means -4 k + b = 0, -2 k + b = 2; 
k = 1, b = 4, y = x+4. Answer: x +4, if x -2 y = if -2 x £ 3 3 if x 3VIII.Knowledge control. So, let's summarize briefly. What did we repeat in the lesson? Plan for studying functions, steps to construct a graph of a piecewise function, specifying a function analytically. Let's check how you have mastered this material. Testing for “4” - “5”, “3” I option No. U
2 1 -1 -1 1 X
- D(f) = , convex up and down on , convex up and down on , decreases on ________ Bounded by ____________ does not exist at all, at most =_____ Continuous throughout the entire domain of definition E(f) = ____________ Convex both down and up at entire definition area
Real processes occurring in nature can be described using functions. Thus, we can distinguish two main types of processes that are opposite to each other - these are gradual or continuous And spasmodic(an example would be a ball falling and bouncing). But if there are discontinuous processes, then there are special means to describe them. For this purpose, functions are introduced that have discontinuities and jumps, that is, in different sections of the number line the function behaves according to different laws and, accordingly, is given by different formulas. The concepts of discontinuity points and removable discontinuity are introduced.
Surely you have already come across functions defined by several formulas, depending on the values of the argument, for example:
y = (x – 3, for x > -3;
(-(x – 3), at x< -3.
Such functions are called piecewise or piecewise specified. Sections of the number line with various formulas tasks, let's call them components domain of definition. The union of all components is the domain of definition of the piecewise function. Those points that divide the domain of definition of a function into components are called boundary points. Formulas that define a piecewise function on each component of the domain of definition are called incoming functions. Graphs of piecewise given functions are obtained by combining parts of graphs constructed on each of the partition intervals.
Exercises.
Construct graphs of piecewise functions:
1)
(-3, at -4 ≤ x< 0,
f(x) = (0, for x = 0,
(1, at 0< x ≤ 5.
The graph of the first function is a straight line passing through the point y = -3. It originates at a point with coordinates (-4; -3), runs parallel to the x-axis to a point with coordinates (0; -3). The graph of the second function is a point with coordinates (0; 0). The third graph is similar to the first - it is a straight line passing through the point y = 1, but already in the area from 0 to 5 along the Ox axis.
Answer: Figure 1.
2)
(3 if x ≤ -4,
f(x) = (|x 2 – 4|x| + 3|, if -4< x ≤ 4,
(3 – (x – 4) 2 if x > 4.
Let's consider each function separately and build its graph.
So, f(x) = 3 is a straight line parallel to the Ox axis, but it needs to be depicted only in the area where x ≤ -4.
Graph of the function f(x) = |x 2 – 4|x| + 3| can be obtained from the parabola y = x 2 – 4x + 3. Having constructed its graph, the part of the figure that lies above the Ox axis must be left unchanged, and the part that lies under the abscissa axis must be symmetrically displayed relative to the Ox axis. Then symmetrically display the part of the graph where
x ≥ 0 relative to the Oy axis for negative x. We leave the graph obtained as a result of all transformations only in the area from -4 to 4 along the abscissa axis.
The graph of the third function is a parabola, the branches of which are directed downward, and the vertex is at the point with coordinates (4; 3). We depict the drawing only in the area where x > 4.
Answer: Figure 2.
3)
(8 – (x + 6) 2, if x ≤ -6,
f(x) = (|x 2 – 6|x| + 8|, if -6 ≤ x< 5,
(3 if x ≥ 5.
The construction of the proposed piecewise given function is similar to the previous paragraph. Here the graphs of the first two functions are obtained from the transformations of the parabola, and the graph of the third is a straight line parallel to Ox.
Answer: Figure 3.
4) Graph the function y = x – |x| + (x – 1 – |x|/x) 2 .
Solution. The scope of this function is all real numbers, except zero. Let's expand the module. To do this, consider two cases:
1) For x > 0 we get y = x – x + (x – 1 – 1) 2 = (x – 2) 2.
2) At x< 0 получим y = x + x + (x – 1 + 1) 2 = 2x + x 2 .
Thus, we have a piecewise defined function:
y = ((x – 2) 2, for x > 0;
( x 2 + 2x, at x< 0.
The graphs of both functions are parabolas, the branches of which are directed upward.
Answer: Figure 4.
5) Draw a graph of the function y = (x + |x|/x – 1) 2.
Solution.
It is easy to see that the domain of the function is all real numbers except zero. After expanding the module, we obtain a piecewise given function:
1) For x > 0 we get y = (x + 1 – 1) 2 = x 2 .
2) At x< 0 получим y = (x – 1 – 1) 2 = (x – 2) 2 .
Let's rewrite it.
y = (x 2, for x > 0;
((x – 2) 2 , at x< 0.
The graphs of these functions are parabolas.
Answer: Figure 5.
6) Is there a function whose graph on the coordinate plane has a common point with any straight line?
Solution.
Yes, it exists.
An example would be the function f(x) = x 3 . Indeed, the graph of a cubic parabola intersects with the vertical line x = a at point (a; a 3). Let now the straight line be given by the equation y = kx + b. Then the equation
x 3 – kx – b = 0 has a real root x 0 (since a polynomial of odd degree always has at least one real root). Consequently, the graph of the function intersects with the line y = kx + b, for example, at the point (x 0; x 0 3).
website, when copying material in full or in part, a link to the original source is required.
Municipal budgetary educational institution
secondary school No. 13
"Piecewise functions"
Sapogova Valentina and
Donskaya Alexandra
Head Consultant:
Berdsk
1. Determination of main goals and objectives.
2. Questionnaire.
2.1. Determining the relevance of the work
2.2. Practical significance.
3. History of functions.
4. General characteristics.
5. Methods for specifying functions.
6. Construction algorithm.
8. Literature used.
1. Determination of main goals and objectives.
Target:
Find out a way to solve piecewise functions and, based on this, create an algorithm for their construction.
Tasks:
Get to know general concept about piecewise functions;
Find out the history of the term “function”;
Conduct a survey;
Identify ways to specify piecewise functions;
Create an algorithm for their construction;
2. Questionnaire.
A survey was conducted among high school students on their ability to construct piecewise functions. The total number of respondents was 54 people. Among them, 6% completed the work completely. 28% were able to complete the work, but with certain errors. 62% were unable to complete the work, although they made some attempts, and the remaining 4% did not start work at all.
From this survey we can conclude that the students of our school who are taking the program do not have a sufficient knowledge base, because this author does not pay attention to special attention for tasks of this kind. It is from this that the relevance and practical significance of our work follows.
2.1. Determining the relevance of the work.
Relevance:
Piecewise functions are found both in the GIA and in the Unified State Exam; tasks that contain functions of this kind are scored 2 or more points. And, therefore, your assessment may depend on their decision.
2.2. Practical significance.
The result of our work will be an algorithm for solving piecewise functions, which will help to understand their construction. And it will increase your chances of getting the grade you want in the exam.
3. History of functions.
“Algebra 9th grade”, etc.;
Analytical function assignment
Function %%y = f(x), x \in X%% is given in an explicit analytical way, if given a formula indicating the sequence of mathematical operations that must be performed with the argument %%x%% in order to obtain the value %%f(x)%% of this function.
Example
- %% y = 2 x^2 + 3x + 5, x \in \mathbb(R)%%;
- %% y = \frac(1)(x - 5), x \neq 5%%;
- %% y = \sqrt(x), x \geq 0%%.
So, for example, in physics, with uniformly accelerated rectilinear motion, the speed of a body is determined by the formula %%v = v_0 + a t%%, and the formula for moving %%s%% of a body with uniform accelerated movement over the time interval from %%0%% to %%t%% is written as: %% s = s_0 + v_0 t + \frac(a t^2)(2) %%.
Piecewise defined functions
Sometimes the function in question can be specified by several formulas that operate in different parts of its domain of definition, in which the argument of the function changes. For example: $$ y = \begin(cases) x ^ 2,~ if~x< 0, \\ \sqrt{x},~ если~x \geq 0. \end{cases} $$
Functions of this type are sometimes called composite or piecewise specified. An example of such a function is %%y = |x|%%
Function Domain
If a function is specified in an explicit analytical way using a formula, but the domain of definition of the function in the form of the set %%D%% is not specified, then by %%D%% we will always mean the set of values of the argument %%x%% for which this formula makes sense. So for the function %%y = x^2%% the domain of definition is the set %%D = \mathbb(R) = (-\infty, +\infty)%%, since the argument %%x%% can take any values on number line. And for the function %%y = \frac(1)(\sqrt(1 - x^2))%% the domain of definition will be the set of values %%x%% satisfying the inequality %%1 - x^2 > 0%%, t .e. %%D = (-1, 1)%%.
Advantages of explicitly specifying a function analytically
Note that the explicit analytical method of specifying a function is quite compact (the formula, as a rule, takes up little space), is easy to reproduce (the formula is not difficult to write) and is most suitable for performing mathematical operations and transformations on functions.
Some of these operations - algebraic (addition, multiplication, etc.) - are well known from school course mathematics, others (differentiation, integration) will be studied in the future. However, this method is not always clear, since the nature of the function’s dependence on the argument is not always clear, and sometimes cumbersome calculations are required to find the function values (if they are necessary).
Implicit function assignment
Function %%y = f(x)%% defined in an implicit analytical way, if the relation $$F(x,y) = 0 is given, ~~~~~~~~~~(1)$$ connecting the values of the function %%y%% and the argument %%x%%. If you specify argument values, then to find the value of %%y%% corresponding to a specific value of %%x%%, you need to solve the equation %%(1)%% for %%y%% at this specific value of %%x%%.
Given the value %%x%%, the equation %%(1)%% may have no solution or have more than one solution. In the first case, the specified value %%x%% does not belong to the domain of definition of the implicitly specified function, and in the second case it specifies multivalued function, which has more than one meaning for a given argument value.
Note that if the equation %%(1)%% can be explicitly resolved with respect to %%y = f(x)%%, then we obtain the same function, but already specified in an explicit analytical way. So, the equation %%x + y^5 - 1 = 0%%
and the equality %%y = \sqrt(1 - x)%% define the same function.
Parametric function specification
When the dependence of %%y%% on %%x%% is not given directly, but instead the dependences of both variables %%x%% and %%y%% on some third auxiliary variable %%t%% are given in the form
$$ \begin(cases) x = \varphi(t),\\ y = \psi(t), \end(cases) ~~~t \in T \subseteq \mathbb(R), ~~~~~ ~~~~~(2) $$what they talk about parametric method of specifying the function;
then the auxiliary variable %%t%% is called a parameter.
If it is possible to eliminate the parameter %%t%% from the equations %%(2)%%, then we arrive at a function defined by the explicit or implicit analytical dependence of %%y%% on %%x%%. For example, from the relations $$ \begin(cases) x = 2 t + 5, \\ y = 4 t + 12, \end(cases), ~~~t \in \mathbb(R), $$ except for the % parameter %t%% we obtain the dependence %%y = 2 x + 2%%, which defines a straight line in the %%xOy%% plane.
Graphic method
Example graphic task functions
The above examples show that the analytical method of specifying a function corresponds to its graphic image, which can be considered as a convenient and visual form of describing a function. Sometimes used graphic method specifying a function when the dependence of %%y%% on %%x%% is specified by a line on the plane %%xOy%%. However, despite all the clarity, it loses in accuracy, since the values of the argument and the corresponding function values can be obtained from the graph only approximately. The resulting error depends on the scale and accuracy of the measurement of the abscissa and ordinate of individual points on the graph. In what follows, we will assign the function graph only the role of illustrating the behavior of the function and therefore will limit ourselves to constructing “sketches” of graphs that reflect the main features of the functions.
Tabular method
Note tabular method function assignments, when some argument values and the corresponding function values are placed in a table in a certain order. This is how the famous tables are built trigonometric functions, tables of logarithms, etc. The relationship between quantities measured in experimental studies, observations, and tests is usually presented in the form of a table.
The disadvantage of this method is that it is impossible to directly determine function values for argument values not included in the table. If there is confidence that the argument values not presented in the table belong to the domain of definition of the function in question, then the corresponding function values can be approximately calculated using interpolation and extrapolation.
Example
| x | 3 | 5.1 | 10 | 12.5 |
| y | 9 | 23 | 80 | 110 |
Algorithmic and verbal methods of specifying functions
The function can be set algorithmic(or software) in a way that is widely used in computer calculations.
Finally, it can be noted descriptive(or verbal) a way to specify a function, when the rule for matching the function values to the argument values is expressed in words.
For example, the function %%[x] = m~\forall (x \in )
